Formulario De Derivadas Completo.pdf

Published July 10, 2023 | By tawnshan

How to Use the Complete Derivatives Form in PDF Format

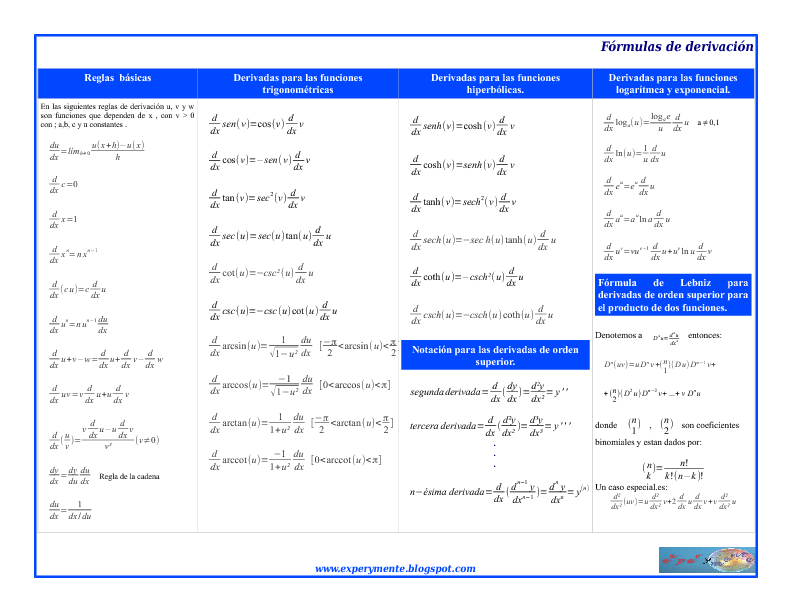
If you are studying calculus, you may have encountered the Formulario De Derivadas Completo.pdf, a document that contains a comprehensive table of derivatives and integration rules. This document can be very useful for solving calculus problems, but how do you use it effectively?

In this article, we will explain what the Formulario De Derivadas Completo.pdf is, how to download it, and how to apply it to different types of functions. We will also give you some tips and tricks to make the most of this valuable resource.

What is the Formulario De Derivadas Completo.pdf?

The Formulario De Derivadas Completo.pdf is a PDF document that contains a table of derivatives and integration rules for various functions. It was created by Arquimedes1075 and uploaded to Google Drive. The document has 12 pages and covers the following topics:

Basic rules of differentiation and integration
Derivatives and integrals of polynomial, exponential, logarithmic, trigonometric, inverse trigonometric, hyperbolic, and inverse hyperbolic functions
Derivatives and integrals of composite functions using the chain rule, the product rule, the quotient rule, and the logarithmic rule
Derivatives and integrals of implicit functions using the implicit differentiation and integration techniques
Derivatives and integrals of parametric functions using the parametric differentiation and integration techniques
Derivatives and integrals of vector functions using the vector differentiation and integration techniques
Derivatives and integrals of special functions such as the absolute value function, the sign function, the Heaviside function, the Dirac delta function, etc.
Some useful formulas and identities involving derivatives and integrals

The document also provides some examples and exercises to help you practice your skills.

How to download the Formulario De Derivadas Completo.pdf?

To download the Formulario De Derivadas Completo.pdf, you can follow these simple steps:

Go to this link: https://drive.google.com/file/d/0B_BF-sscN85KSEtGbG02R3hvbU0/view
Click on the download icon at the top right corner of the screen.
Select a location on your computer where you want to save the file.
Open the file with a PDF reader such as Adobe Acrobat Reader or Foxit Reader.

You can also print the document if you prefer to have a hard copy.

How to apply the Formulario De Derivadas Completo.pdf to different types of functions?

To apply the Formulario De Derivadas Completo.pdf to different types of functions, you need to identify the type of function you are dealing with and then look for the corresponding rule in the table. For example:

Example 1: Find the derivative of f(x) = x^3 + 2x – 5.

This is a polynomial function, so we can use the rule for derivatives of polynomials:

$$f'(x) = \frac{d}{dx}(x^3 + 2x – 5) = \frac{d}{dx}(x^3) + \frac{d}{dx}(2x) – \frac{d}{dx}(5)$$

Using the power rule, we get:

$$f'(x) = 3x^2 + 2 – 0$$

Simplifying, we get:

$$f'(x) = 3x^2 + 2$$

Example 2: Find the integral of f(x) = sin(x).

This is a trigonometric function, so we can use the rule for integrals of trigonometric functions:

$$\int f(x) dx = \int sin(x) dx$$

Using the antiderivative of sin(x), we get:

$$\int f(x) dx = -cos(x) + C$$

Where C is an arbitrary constant.

Example 3: Find the derivative of f(x) = ln(x^2 + 1).

This is a composite function, so we can use the chain rule:

$$f'(x) = \frac{d}{dx}(ln(x^2 + 1)) = \frac{1}{x^2 + 1} \cdot \frac{d}{dx}(x^2 + 1)$$

Using the power rule, we get:

$$f'(x) = \frac{1}{x^2 + 1} \cdot (2x)$$

Simplifying, we get:

$$f'(x) = \frac{2x}{x^2 + 1}$$

How to use the Formulario De Derivadas Completo.pdf for integration by parts?

Integration by parts is a technique that allows us to integrate products of functions that are not easily integrable. The formula for integration by parts is:

$$\int u dv = uv – \int v du$$

Where u and v are functions of x, and du and dv are their respective differentials. To use this formula, we need to choose u and dv wisely, so that the resulting integral is simpler than the original one. The Formulario De Derivadas Completo.pdf can help us with this choice, as it provides a list of common choices for u and dv in different situations. For example:

Example 4: Find the integral of f(x) = x ln(x).

This is a product of a polynomial function and a logarithmic function, so we can use the rule for integration by parts of polynomials and logarithms:

$$\int x ln(x) dx = \frac{x^2}{2} ln(x) – \frac{x^2}{4} + C$$

Where C is an arbitrary constant. To get this result, we chose u = ln(x) and dv = x dx, and then applied the formula.

How to use the Formulario De Derivadas Completo.pdf for integration by substitution?

Integration by substitution is a technique that allows us to transform a complicated integral into a simpler one by changing the variable of integration. The formula for integration by substitution is:

$$\int f(g(x)) g'(x) dx = \int f(u) du$$

Where u = g(x) is a new variable, and du = g'(x) dx is its differential. To use this formula, we need to find a suitable substitution that makes the integral easier to solve. The Formulario De Derivadas Completo.pdf can help us with this task, as it provides a list of common substitutions for different types of functions. For example:

Example 5: Find the integral of f(x) = (x^2 + 1)^3.

This is a composite function of a polynomial function, so we can use the rule for integration by substitution of polynomials:

$$\int (x^2 + 1)^3 dx = \frac{(x^2 + 1)^4}{8} + C$$

Where C is an arbitrary constant. To get this result, we chose u = x^2 + 1 and du = 2x dx, and then applied the formula.

How to use the Formulario De Derivadas Completo.pdf for integration by partial fractions?

Integration by partial fractions is a technique that allows us to integrate rational functions, that is, functions that are quotients of polynomials. The idea is to decompose the rational function into simpler fractions that are easier to integrate. The Formulario De Derivadas Completo.pdf can help us with this process, as it provides a list of common cases and formulas for integration by partial fractions. For example:

Example 6: Find the integral of f(x) = \frac{1}{x^2 – 1}.

This is a rational function with a quadratic denominator, so we can use the rule for integration by partial fractions of quadratic denominators:

$$\int \frac{1}{x^2 – 1} dx = \frac{1}{2} \int \frac{1}{x – 1} dx – \frac{1}{2} \int \frac{1}{x + 1} dx$$

To get this result, we decomposed the rational function into two simpler fractions using the method of equating coefficients. Then, we integrated each fraction using the rule for integrals of rational functions:

$$\int \frac{1}{x^2 – 1} dx = \frac{1}{2} ln|x – 1| – \frac{1}{2} ln|x + 1| + C$$

Where C is an arbitrary constant.

How to use the Formulario De Derivadas Completo.pdf for integration by trigonometric substitution?

Integration by trigonometric substitution is a technique that allows us to integrate functions that involve square roots of quadratic expressions. The idea is to replace the variable of integration with a trigonometric function that simplifies the expression under the square root. The Formulario De Derivadas Completo.pdf can help us with this choice, as it provides a list of common trigonometric substitutions for different cases. For example:

Example 7: Find the integral of f(x) = \frac{1}{\sqrt{x^2 + 4}}.

This is a function that involves a square root of a quadratic expression with a positive constant term, so we can use the rule for integration by trigonometric substitution of positive constant terms:

$$\int \frac{1}{\sqrt{x^2 + 4}} dx = \int \frac{sec(\theta)}{2} d\theta$$

To get this result, we chose x = 2 tan(\theta) and dx = 2 sec^2(\theta) d\theta, and then applied the Pythagorean identity. Then, we integrated the resulting function using the rule for integrals of trigonometric functions:

$$\int \frac{1}{\sqrt{x^2 + 4}} dx = \frac{sec(\theta)}{2} + C$$

Where C is an arbitrary constant. To get the final answer in terms of x, we used the inverse trigonometric function:

$$\int \frac{1}{\sqrt{x^2 + 4}} dx = \frac{\sqrt{x^2 + 4}}{2} + C$$

How to use the Formulario De Derivadas Completo.pdf for integration by trigonometric identities?

Integration by trigonometric identities is a technique that allows us to integrate functions that involve trigonometric functions. The idea is to use some formulas and identities that relate different trigonometric functions and simplify the integrand. The Formulario De Derivadas Completo.pdf can help us with this task, as it provides a list of common trigonometric identities and formulas for integration by trigonometric identities. For example:

Example 8: Find the integral of f(x) = sin^3(x) cos^2(x).

This is a function that involves powers of trigonometric functions, so we can use the rule for integration by trigonometric identities of powers:

$$\int sin^3(x) cos^2(x) dx = \int \frac{sin(2x)}{4} – \frac{sin^4(2x)}{8} dx$$

To get this result, we used the identity sin^2(x) = \frac{1 – cos(2x)}{2} and the double angle formula sin(2x) = 2 sin(x) cos(x), and then expanded and simplified the integrand. Then, we integrated each term using the rule for integrals of trigonometric functions:

$$\int sin^3(x) cos^2(x) dx = -\frac{cos(2x)}{8} + \frac{cos^4(2x)}{32} + C$$

Where C is an arbitrary constant.

How to use the Formulario De Derivadas Completo.pdf for integration by reduction formulas?

Integration by reduction formulas is a technique that allows us to integrate functions that involve repeated integrations of the same function or its derivatives. The idea is to use a formula that relates the integral of a function with a lower power or order to the integral of the same function with a higher power or order. The Formulario De Derivadas Completo.pdf can help us with this technique, as it provides a list of common reduction formulas for different types of functions. For example:

Example 9: Find the integral of f(x) = x^n e^x.

This is a function that involves a product of a polynomial function and an exponential function, so we can use the rule for integration by reduction formulas of polynomials and exponentials:

$$\int x^n e^x dx = x^n e^x – n \int x^{n-1} e^x dx$$

To get this result, we used integration by parts with u = x^n and dv = e^x dx, and then applied the formula recursively until we reached a simpler integral. Then, we integrated the final term using the rule for integrals of exponential functions:

$$\int x^n e^x dx = x^n e^x – n x^{n-1} e^x + n(n-1) x^{n-2} e^x – … + (-1)^n n! e^x + C$$

Where C is an arbitrary constant.

Conclusion

In this article, we have shown you how to use the Formulario De Derivadas Completo.pdf, a comprehensive table of derivatives and integration rules, for solving calculus problems. We have explained what the document is, how to download it, and how to apply it to different types of functions. We have also given you some examples and exercises to help you practice your skills.

The Formulario De Derivadas Completo.pdf is a valuable resource for anyone who wants to learn calculus or refresh their knowledge. It can help you save time and avoid mistakes when performing calculations. However, it is not a substitute for understanding the concepts and principles behind calculus. You should always try to understand why a rule works and how to apply it correctly.

We hope you have found this article useful and informative. If you have any questions or feedback, please let us know in the comments section below. Thank you for reading!

86646a7979

Posted in Moonreaderman |

Tagged Formulario De Derivadas Completo.pdf |